???????
— ?????? ??? ??????????? ?????
???????????
. ??? ????? ???????? ??? ???????????, ??????????? ?????????. ???????? ?????
?????? ???????????
????????
??????????
?
????????? ????? ?????????
. ???????????? ???? ????????? ?? ???????? ?????????? ? ??????? ??????, ??? ??? ???????????? ?? ???????? ?? ????????
???????
. ???? ?? ???????? ?????????? ???????? ?????????-????????????? ????????? — ?????????? ?
ZFC
??????????? ?? ??? ??????? ? ?????????????
.
??????? ???????? ?????? ????????????? ?
?????????????? ?????????
, ? ???????
?????????????? ?????????????
??????????????
??????????????
??????? (????????,
??????????????? ??????
), ?
??????????? ????????????
—
????????????
????? ????? ?????????. ???????????? ???????? ???????? ??????????????? ?? ?????? ???????? ?????????? ? ???????????? ??? ????, ????? ????????? ????? ????? ????????? ?????????.
?????? «???????» ??? ????????????? ???????????? ?? ????? ????????
???????? ???????
, ??? ???? ? ??????? ????? «???????» ?????????? ? ???????????????? ???????
.
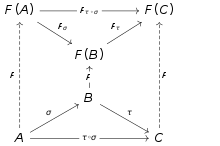
???????????
???????
F
{\displaystyle F}
?????? ????????? ?????????? ?????????
?
{\displaystyle \tau }
?
?
{\displaystyle \sigma }
(????????????) ???????
F
:
C
?
D
{\displaystyle {\mathcal {F}}\colon {\mathcal {C}}\to {\mathcal {D}}}
??
?????????
C
{\displaystyle {\mathcal {C}}}
? ?????????
D
{\displaystyle {\mathcal {D}}}
— ??? ???????????, ???????:
???????????? ??????? ???????
X
?
C
{\displaystyle X\in {\mathcal {C}}}
??????
F
(
X
)
?
D
,
{\displaystyle {\mathcal {F}}(X)\in {\mathcal {D}},}
???????????? ???????
????????
f
:
X
?
Y
{\displaystyle f:X\to Y}
? ?????????
C
{\displaystyle {\mathcal {C}}}
???????
F
(
f
)
:
F
(
X
)
?
F
(
Y
)
{\displaystyle {\mathcal {F}}(f):{\mathcal {F}}(X)\to {\mathcal {F}}(Y)}
? ?????????
D
{\displaystyle {\mathcal {D}}}
. ??? ????????????? ?????? ???????? ?????????? ??????????:
F
(
i
d
A
)
=
i
d
F
(
A
)
{\displaystyle {\mathcal {F}}(\mathrm {id} _{A})=\mathrm {id} _{{\mathcal {F}}(A)}}
,
F
(
g
?
f
)
=
F
(
g
)
?
F
(
f
)
{\displaystyle {\mathcal {F}}(g\circ f)={\mathcal {F}}(g)\circ {\mathcal {F}}(f)}
.
????? ???????, ??????? ?????? ????????? ????????????? ???????? ? ????????? ?????????? ?????????.
??????????? ???????,
???????????????? ???????
— ??? ???????????, ?????????? ??????? (?? ???? ?????????????? ????????
f
:
X
?
Y
{\displaystyle f:X\to Y}
???????
F
(
f
)
:
F
(
Y
)
?
F
(
X
)
{\displaystyle {\mathcal {F}}(f):{\mathcal {F}}(Y)\to {\mathcal {F}}(X)}
), ??????????? ????????????? ???????? ? ??????????????? ?????????:
F
(
g
?
f
)
=
F
(
f
)
?
F
(
g
)
{\displaystyle {\mathcal {F}}(g\circ f)={\mathcal {F}}(f)\circ {\mathcal {F}}(g)}
.
????? ???????????????? ??????? ????? ?????????? ??? ???????????? ??????? ??
???????????? ?????????
C
o
p
{\displaystyle {\mathcal {C}}^{\mathrm {op} }}
. ????????? ?????? ???????????? ?????????? ??? ????????? ???????????, ? ?????? ???? «???????????????? ??????? ??
C
{\displaystyle {\mathcal {C}}}
?
D
{\displaystyle {\mathcal {D}}}
» ??????? «??????? ??
C
o
p
{\displaystyle {\mathcal {C}}^{\mathrm {op} }}
?
D
{\displaystyle {\mathcal {D}}}
» (???, ??????, «??????? ??
C
{\displaystyle {\mathcal {C}}}
?
D
o
p
{\displaystyle {\mathcal {D}}^{\mathrm {op} }}
»).
?????????? ? ??????????????
?????????
— ??? ??????? ?? ???? ??????????. ???????????? ?????? —
??????? Hom
, ?? ??????????? ?? ?????? ????????? ? ??????????????? ?? ???????.
????????? ?????????? ???????????? ??? ???????? ??
????????? ????????????
. ????????, ???????
H
o
m
{\displaystyle \mathrm {Hom} }
????? ???
C
o
p
×
C
?
S
e
t
{\displaystyle {\mathcal {C}}^{\mathrm {op} }\times {\mathcal {C}}\to \mathbf {Set} }
.
?????????????
— ??? ????????? ??????? ?????????? ??
n
{\displaystyle n}
??????????.
???????
??? ??????? ???????? ????? ?????????? ???????? ??? ?? ?????? ?? ???????? ?????????, ?? ? (??? ????? ?????) ?? ?????????: ?????????? ????????? ????????, ??????????? ????????? ?? ????????, ????????,
????????????? ???????
?
????????????????? ???????
, ?????????? ???????.
?????
C
{\displaystyle {\mathcal {C}}}
—
????????????
? ?????????
D
{\displaystyle {\mathcal {D}}}
. ? ????? ?????? ?????????
??????? ????????
I
:
C
?
D
{\displaystyle I:{\mathcal {C}}\hookrightarrow {\mathcal {D}}}
, ??????????? ?? ???????? ? ????????? ??? ???????????????
????????
???????.
?????????? ???????:
???????, ???????????? ?????? ?????? ?????????
C
{\displaystyle {\mathcal {C}}}
? ????????????? ?????? ?????????
D
{\displaystyle {\mathcal {D}}}
, ? ?????? ???????
C
{\displaystyle {\mathcal {C}}}
— ? ????????????? ??????? ????? ???????.
??????????????
???????? ????? ???????? ?? ????????? ? ????.
???????????? ????????? ????????????
: ???????????, ?????????????? ???????
?????????? ????????????
????????????
? ????, ? ???????
????????? ???????????
— ???????????? (??? ?????????????????) ???????????, ???????? ???????????????? ????????????? ?? ????????? ????????? ???????????.
?????
C
{\displaystyle {\mathcal {C}}}
—
?????????? ?????????
, ?? ???? ?????????, ??????????
???????????? ?????????
?
????????? ????????
(??????? ??????
??????????? ????????
). ? ??????? ????? ???????? ???????? ????????? ????????????? ?????????, ? ????? ?????? ? ?????????, ??? ? ???????? ?? ???? ??????????, ??????????? ?????????????? ????????? (??????:
????????? ?????
,
????????? ?????
,
????????? ????????
).
????? ???????????
(???? ?? ??????????) ? ??????????? ???????? ???? ??????? ?????????? ??????? (??????:
????????? ??????
).
?????????
: ?????
X
{\displaystyle X}
—
?????????????? ????????????
, ?????
???????? ????????????
X
{\displaystyle X}
????????
???????? ????????????? ?????????
?? ????????? ?????????, ????????????
O
(
X
)
{\displaystyle O(X)}
. ??? ? ?????? ???????? ?????????????? ?????????,
O
(
X
)
{\displaystyle O(X)}
????? ??????????? ?????????, ???????? ???????????? ???????
U
?
V
{\displaystyle U\to V}
????? ? ?????? ?????, ?????
U
?
V
{\displaystyle U\subseteq V}
. ???????????????? ???????? ??
O
(
X
)
{\displaystyle O(X)}
??????????
???????????
. ????????, ?????????? ??????? ? ?????????
??????????????
??????
, ?????????????? ????????? ????????? ??????? ?????????????????? ??????????? ??????? ?? ???.
??????????????? ??????
: ??????? ??????????????? ????????????
X
{\displaystyle X}
? ?????????? ??????
x
0
{\displaystyle x_{0}}
????? ???????????
??????????????? ??????
?
1
(
X
,
x
0
)
{\displaystyle \pi _{1}(X,x_{0})}
, ???????? ??????? — ?????? ??????????????? ?????? ? ????????? ??
?????????
. ????
f
:
X
?
(
Y
)
{\displaystyle f:X\to (Y)}
— ??????? ??????????? ? ?????????? ?????? (??????????? ???????????, ??????????? ?????????? ????? ??????? ???????????? ? ?????????? ????? ???????), ?????? ????? ?? ?????
x
0
{\displaystyle x_{0}}
????? ??????????? ?? ?????, ?????????? ?????? ?? ?????
y
0
{\displaystyle y_{0}}
. ??? ????????????? ??????????? ? ???????? ??????????????? ? ? ????????? ??????????, ?????????????, ????????
?????????????
??
?
(
X
,
x
0
)
{\displaystyle \pi (X,x_{0})}
?
?
(
Y
,
y
0
)
{\displaystyle \pi (Y,y_{0})}
. ???????? ?????????, ??? ??????????? ? ??? ????????? ???????? ????????????? ???????? ?? ????????? ?????????????? ??????????? ? ?????????? ?????? ?
????????? ?????
.
??????????? ? ????????????? ??????????
: ???????????, ??????????????
???????? ????????????
???
??????????? ??????????
, ? ?????????????? ???????????? — ???
????????????
, ???????? ???????????? ????????? ?? ????????? ??????? ???????????? ? ??????????????? ? ?????????
????????? ??????????
. ??????????,
????????????? ??????????
?
??????????????
?????????????? ?????? ???????????????? ???????.
???????????? ???????????? ???????????? ? ????????????? ????? ?????? ???????????? ??????? ?? ????????? ??????? ???????????? ? ?????????? ?????? ? ??????? ??????????? ? ????????? ????????? ???????????.
????????? ????????????
: ????
C
{\displaystyle {\mathcal {C}}}
— ????????? ????????? ??????????? ??? ????????????? ?????,
????????? ????????????
???? ??????????? ?????? ???????
C
×
C
?
C
{\displaystyle {\mathcal {C}}\times {\mathcal {C}}\to {\mathcal {C}}}
, ???????????? ?? ????? ??????????
.
— ???????????? ???????????????? ???????? ??
?????????????? ?????????
? ????????? ????????? (?
????????? ????????
—
?????????????? ?????????
, ?
????????? ?????
—
? ??????); ???????????, ?????????? ???????
??????????????? ?????????
, ?????? ?????? ???? ? ?????????????? ?????????.
???????
Gal
:
F
l
d
o
p
?
G
r
p
{\displaystyle \operatorname {Gal} :\mathbf {Fld} ^{\mathrm {op} }\to \mathbf {Grp} }
????????????
????
F
{\displaystyle F}
???
?????????? ?????? ?????
Gal
?
(
F
¯
/
F
)
{\displaystyle \operatorname {Gal} ({\bar {F}}/F)}
, ? ???????????? ????? — ???????????????
[
?????????
]
??????????? ????? ?????.
????????
??????? ?????????
????????????? ?????????
? ????????????? ?????????.
??????? ????????? ??????????? ? ???????????.
?????????? ???? ????????? ???? ???????? ?????????. ?????????? ????????? ????????
????????????? ?????????
(???, ??? ??? ??????????), ??????? ???????? ????? ?????? ??????????? ????????????? ???? ?????????
?????????
? ?????????.
????????? ?? ?????? ??????? — ?? ?? ?????, ???
??????
: ???????? ? ??? ????????????? ????????? ???????, ? ???????? ?????????? ????????? — ????????, ???????????? ? ???????. ???????? ????? ??????????? ? ????? ???????? ??????? ?????????? ????????????? ????????????? ????????; ?????????????, ? ????????? ?????? ??????? ???????? ?????????? ??????? ???????????? ???????? ?? «???????, ? ??????? ???????? ?????????? ?????????? ?? ?????».
????? ? ??????? ??????????? ?????????
?????
C
{\displaystyle {\mathcal {C}}}
?
D
{\displaystyle {\mathcal {D}}}
— ?????????. ????????? ???? ?????????
F
:
C
?
D
{\displaystyle {\mathcal {F}}\colon {\mathcal {C}}\to {\mathcal {D}}}
????? ??????? ?????????? ???????? ?????? ?????????:
????????? ?????????
. ???????? ? ???? ????????? —
???????????? ??????????????
?????????.
???????? ???????? ????? ?????? ??? ??????
????????????? ???????
, ??????? ???????? ? ????
????????? ????????????
,
????????????
?????, ???????? ??? ????????? ???????????,
??????
?
????????
???????. ????? ????????????? ??????????? ????? ?????? ????
??????????? ?????????
.
??????????
, ?. 42.
Carnap R.
The Logical Syntax of Language. — Routledge & Kegan Paul, 1937. — P. 13—14.
Hazewinkel M., Gubareni N. M., Kirichenko V. V. .
. — Dordrecht:
Springer Science & Business Media
, 2004. — 380 p. — (Mathematics and Its Applications, vol. 575). —
ISBN 978-1-4020-2690-4
.
— P. 99—100.
??????????
????? ?., ?????? ?. .
???????? ? ?????? ????????? ? ?????????. —
?.
:
???
, 1972. — 259 ?.
??????? ?.
.
????? 2. ??????????? ? ??????????
// ????????? ??? ??????????? ??????????. —
?.
:
?????????
, 2004. — 352 ?. —
ISBN 5-9221-0400-4
.
— ?. 43—67.
??????? ?. ?., ?????????? ?. ?. .
?????? ?????? ?????????. —
?.
:
?????
, 1974. — 256 ?.
??????
Marquis, Jean-Pierre.
(????.)
. Stanford Encyclopedia of Philosophy. — ???????? ? ???? ????? ?????? ?????? ??????????. ???? ?????????: 30 ???? 2013.
13 ??????? 2013 ????.